5) A different concept for "Dimensions"
- andrelefebvre
- 25 mars 2020
- 2 min de lecture
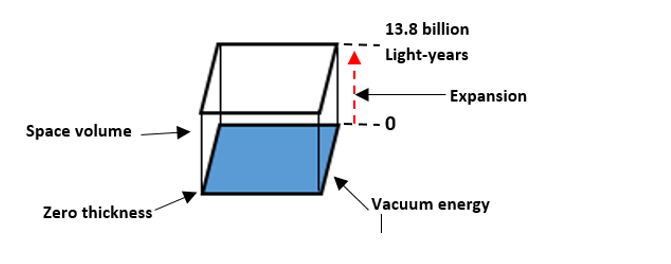
This first dimension, by rotating, will start to expand in a surface (2nd dimension) due to the centrifugal “effect”. This expansion cannot be applied to a “volume”; it starts from an extending non-observable unidimensional (punctual) “point”, which “creates” a bi-dimensional “surface” observable on strictly two faces (it has no “thickness”.
Let me precise the event.
A “zero point” is a non-existing point. You cannot see it because it is not there; it’s only a potentiality of “being there” eventually.
The other reason is that you can never “see” energy anyway; all you can observe is its “effect” (its work). So even if we consider that there’s a bit of energy, it cannot be observed. This non-observed energy is present, even before the geometrical “punctual” point appears, which is the first dimension of our “spatial” (3D) geometry that will appear far later.
So, even if we cannot see it, we will consider it as a non-existing “point” that starts rotating.
In order to “see” what happens through the process, let’s imagine that you are making a pizza. If you’re good at it, you will throw the “pasta” in the air to enlarge it and make it perfectly round. It is the “centrifugal effect” that expands evenly your rotating pizza dough.
And while expanding, the circumference of your pizza will increase its speed which will increase its diameter.
Now, for our mathematics buffs, here is how to calculate the tangential speed:
First you take the diameter and multiply it by “pi”; which is 3.1416 (approximately)
So if you have a diameter of 5 inches the circumference will be 5 x 3.1416 = 15.708 inches.
Then you divide the circumference by the time it takes for one complete rotation. We had 10 seconds in our former drawing; so 15.708 inches divided by 10 seconds = a speed of 1.5708 inches by second.
Therefore when your pizza expands to 10 inches, you get 10 x 3.1416 = 31.416 inches of circumference; divide by 10 seconds = 3.1416 inches per seconds manifested at the circumference.
Has you can see, the speed of the pizza’s circumference doubled while its diameter doubled. Thus there really is an increase of energy supplied by the rotation while the “centrifugal effect” increases the diameter.
But even this speed increase isn’t as fast as the speed increase during the Planck era. The difference is that the quantity of your pizza dough doesn’t change while rotating; which is not the case of our starting bit of energy.
When we have that bit of energy that starts rotating, it doesn’t disappear after the first rotation, but keeps doing its kinetic “action” (its effect still “acts”). So when the second rotation starts, the increase of kinetic energy produced by the rotation, adds itself to the initial starting bit of energy; otherwise the rotation would stop.



Commentaires